Research on the influence of roadway obstacles on the position of wind speed monitoring
-
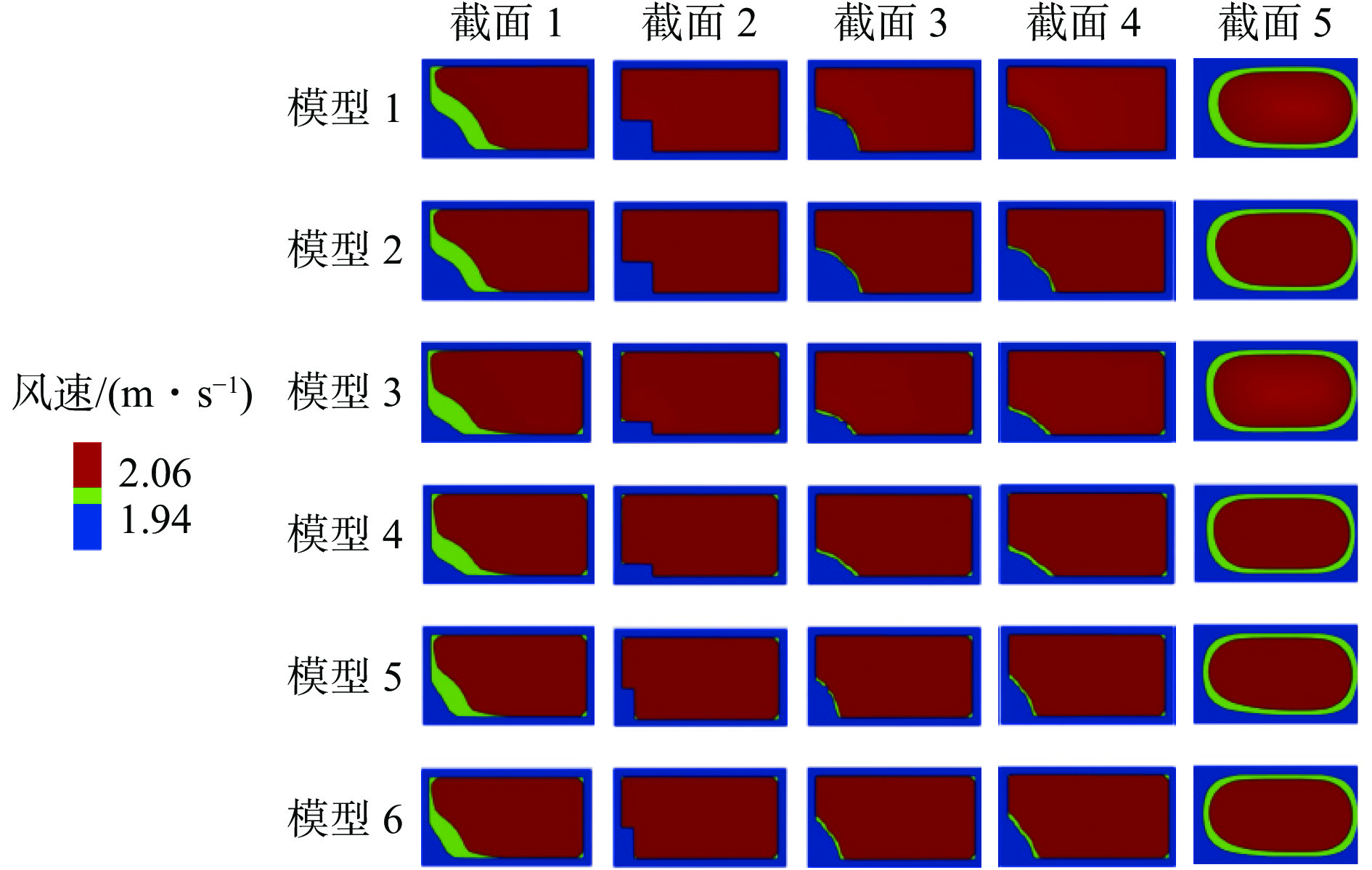
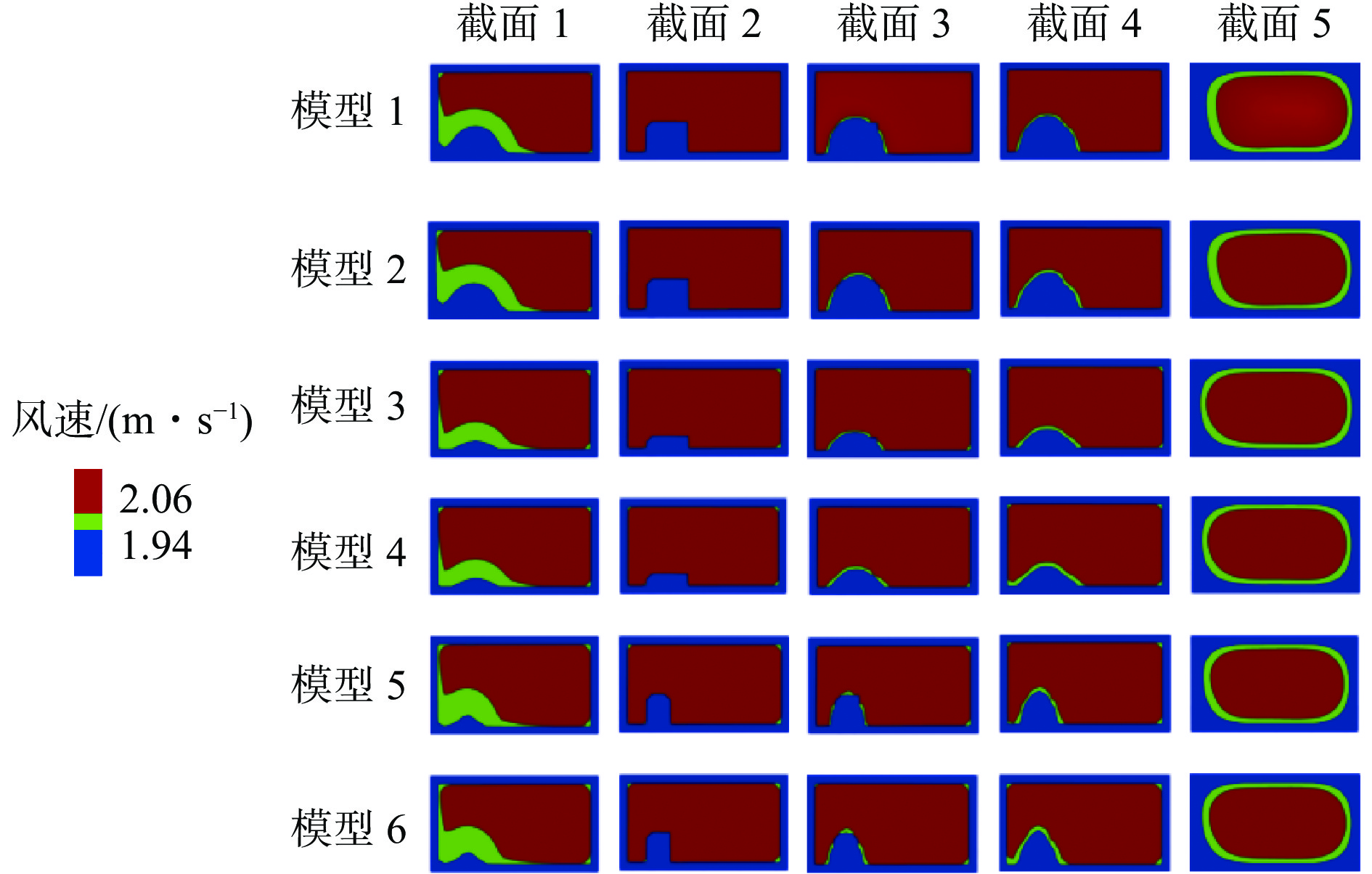
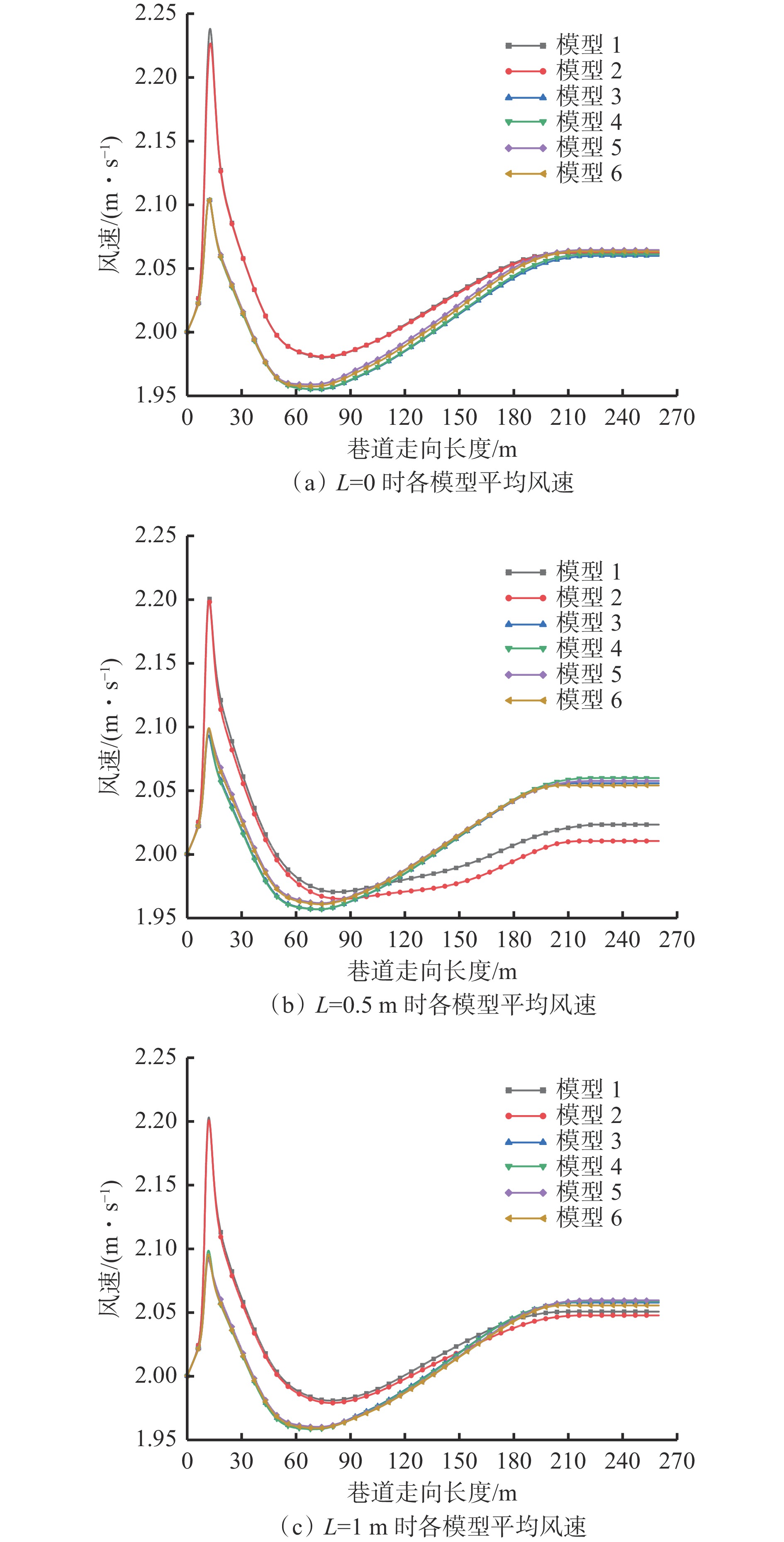
摘要: 现有高精度风速传感器在井下的安装位置统一采用正常风流流动状况下的方案，未综合考虑巷道放置障碍物等导致风流异常的情况，达不到智能通风的风速精度要求，难以实现矿井的安全生产。针对上述问题，以小纪汗煤矿11218回风巷为研究对象，对井下巷道中障碍物不同位置与不同尺寸对风速的影响展开研究，结合现场实测巷道基础参数与Fluent软件构建贴合该矿特征的巷道模型，研究了距上游端口10 m处底板放置的障碍物与两帮的距离（简称间距L）及其形状大小、放置方式等因素对巷道风速监测位置的影响。① 定量分析结果发现：各模型于断面直角处存在微小部分的合理风速区域，其面积在L=0.5 m时最大，L=1 m时次之，L=0时最小；随着间距L的增加，风速传感器最佳布设位置随x坐标（巷道走向）的增加呈均匀分布−截面直角处微量分布−空心圆角矩形分布的规律，且合理风流向两帮扩散更快；L=0时，顶板位置中垂线的合理风流分布在2.59～2.78 m处；L=0.5 m时，顶板位置中垂线的合理风流分布在2.59～2.80 m处；L=1 m时，顶板位置中垂线的合理风流分布在2.61～2.78 m处。② 定性分析结果表明：放置障碍物巷道的平均风速均呈增大−减小−增大−平衡的状态；障碍物竖放或宽度增加对风流影响较大；障碍物体积相同，风速峰值大致相同；风流发展稳定时，L=0.5 m时风速可靠性最高，L=1 m时次之，L=0时可靠性相对最低。③ 通过风速普适性分析得出：在同模型下，不同风速变化率均处于上升−下降−再上升−平衡的4个阶段；在模型2、间距L=0.5 m条件下，对回风巷风流运移规律影响较小的结论具有风速普适性。Abstract: The existing high-precision wind speed sensors are uniformly installed in the coal mines under normal airflow conditions. It does not consider the abnormal airflow caused by obstacles placed in the roadway. It cannot meet the wind speed precision requirements of intelligent ventilation and it is difficult to achieve safe production in the mine. In order to solve the above problems, taking the 11218 return air roadway of Xiaojihan Coal Mine as the research object, the influence of different positions and sizes of obstacles in the underground roadway on wind speed is studied. Based on on-site measured roadway basic parameters and Fluent software, a roadway model is constructed that fits the features of the mine. The influence of factors such as the distance between the obstacle placed on the floor at a distance of 10 meters from the upstream port and the two sides (referred to as the distance L), its shape, size, and position on the monitoring position of roadway wind speed is studied. ① The quantitative analysis results show that there are small reasonable wind speed regions at the right angles of the cross-section for each model. The maximum area is when L=0.5 m, followed by when L=1 m, and the minimum area is when L=0 m. As the distance L increases, the optimal placement position of the wind speed sensor follows a uniform distribution with the increase of the x-coordinate (roadway direction) - a trace distribution at the right angle of the cross-section - a hollow rounded rectangle distribution pattern. The reasonable airflow diffuses faster towards the two sides. When L=0 m, the reasonable airflow distribution of the vertical line in the roof position is at 2.59-2.78 m. When L=0.5 m, the reasonable airflow distribution of the vertical line in the roof position is between 2.59-2.80. When L=1 m, the reasonable airflow distribution of the vertical line in the roof position is 2.61-2.78 m. ② The qualitative analysis results indicate that the average wind speed in the roadway with obstacles is in a state of increase - decrease - increase - balance. The vertical placement or increase in width of obstacles has a significant impact on wind flow. The volume of obstacles is the same, and the peak wind speed is roughly the same. When the wind flow develops steadily, the wind speed reliability is highest at L=0.5 m, followed by L=1 m, and the reliability is lowest at L=0 m. ③ Through the analysis of wind speed universality, it can be concluded that under the same model, different wind speed change rates are in four stages of ascending - descending - ascending - balancing. Under the condition of model 2 and spacing L=0.5 m, the conclusion that the influence on the air flow transport law of the return air roadway is relatively small has wind speed universality.
-
0. 引言
在厚硬顶板沿空留巷条件下，受掘进和采动影响，巷道底板变形，出现底鼓。随着工作面推进，基本顶在采空区上方形成悬顶[1]。一旦发生顶板旋转下沉，会对煤岩体和巷旁支护造成冲击挤压[2]，加剧巷道底板变形[3]。底鼓现象造成巷道断面减小，阻碍设备运输、人员行走，影响工作面正常回采，增加大量维修工作和维护费用。切顶卸压技术可以在巷道采空区一侧形成预裂切缝面[4]，切断采空区和巷道顶板之间的联系，改善巷道围岩结构，减少底板变形量。因此，根据切顶卸压前后的围岩结构变化进一步研究巷道底鼓的变形机理具有一定意义。
针对沿空留巷切顶卸压的底板变形问题，学者们进行了大量研究。康志鹏等[5]以厚煤层软底留巷为背景，提出对底板进行合适的让压，以实现巷道柔性支护。于光远等[6]提出通过“切顶卸压+柔性让压+补强锚索控顶+双控锚杆控帮”的方式控制底鼓，取得了较好的效果。何满潮等[7]针对深部高应力复合破碎顶板切顶留巷，设计了一种新型可缩U型钢挡矸结构，可有效控制巷道底板变形。张幼振[8]提出了巷道底鼓机械化治理的技术思路和机械化施工系统的配置原则，研发了巷道修复机、底板锚固钻机等专用主导机械设备，可有效治理巷道底鼓。申斌学等[9]提出了切顶卸压柔模墙支护沿空留巷技术，在切顶后使用该技术可以对巷道顶板进行强力支护，抑制巷道底鼓。张宇旭等[10]通过试验证明了底板注浆与锚杆锚索联合支护可以改善底板应力状态，控制底板位移。以上有关巷道底鼓研究与实践主要探讨巷道底板的变形机理及控制技术，对沿空留巷切顶卸压前后底板力学分析不全面。针对上述问题，本文建立了巷道围岩和底板力学模型，分析各区域对巷道底板的作用，并推导了沿空留巷切顶前后巷道底鼓变形量计算公式。
1. 工程背景
以淮南某矿沿空留巷工作面为研究对象，工作面长210 m，走向长1 784 m，1号煤厚3.5～8.5 m，平均厚度为6.5 m。煤层倾角为6～8°，平均值为7°。工作面煤层直接顶主要为泥岩，平均厚1 m；基本顶主要为中细砂岩，平均厚13.6 m；直接底主要为泥岩，平均厚0.5 m；基本底主要为砂质泥岩，平均厚3.0 m。工作面布置及岩性特征如图1所示。
![]()
图 1 沿空留巷工作面布置及岩性特征Figure 1. Arrangement of gob-side entry retaining working face and lithological characteristics2. 巷道底鼓力学分析
2.1 沿空留巷围岩结构
工作面开挖后，因直接顶极薄且强度较低，采空区直接顶垮落，基本顶在实体煤体侧断裂。巷旁支护体在顶板载荷作用下产生变形，压缩下沉。此时顶板处于给定变形状态[11]，如图2所示，巷旁支护体主要承受直接顶及悬露基本顶岩块B的重力。上覆岩层破断岩块A，B，C三者相互铰接形成承压结构[12]，承载上覆岩层压力并控制下方巷道的围岩稳定。岩块C下沉后，在上覆岩层载荷作用下与矸石接触发生挤压，并逐渐被压实[13]。
2.2 围岩结构力学分析
在给定变形条件下，侧向实体煤承担围岩应力峰值，巷旁支护体无法有效控制基本顶，岩块B发生旋转下沉。对沿空留巷顶板力学模型作如下假设：① 基本顶于实体煤侧弹塑性交界处断裂，绕直接顶向采空区侧旋转倾斜。② 采空区矸石对岩块C产生支撑力，对岩块B支撑力为0[14]。③ 忽略直接顶、基本顶及更上位岩层之间的剪切力。④ 基本顶上覆岩层载荷均匀施加在基本顶上。⑤ 巷内支护的变形忽略不计[15]。根据假设条件，建立沿空留巷力学模型，如图3所示。
基本顶沿实体煤弹塑性区交界处上方$ {A}' $点断裂并向采空区旋转，煤体极限平衡区宽度x0[16]及塑性区煤体对顶板的支撑力σ分别为
$$ {x_0} = \dfrac{{{h_{\rm{c}}}\lambda }}{{2\tan\; \varphi }}\ln\;{\dfrac{{k\gamma {H} + {c}/{{\tan\; \varphi }}}}{{{c}/{{\tan\; \varphi }} + {{{p_{\rm{x}}}}}/{\lambda }}}} $$ (1) $$ \sigma = \left( {\dfrac{c}{{\tan\; \varphi }} + \dfrac{{{p_{\rm{x}}}}}{\lambda }} \right){\exp\left({\dfrac{{2x_0\tan\; \varphi }}{{{h_{\rm{c}}}\lambda }}}\right)} - \dfrac{c}{{\tan\; \varphi }} $$ (2) 式中：hc为煤层厚度；λ为煤体侧压系数；φ为煤层界面内摩擦角；k为煤帮侧应力集中系数；γ为岩层平均容重；H为开采深度；c为煤层界面黏聚力；px为煤帮侧向支护阻力。
基本顶沿倾向垮落长度L与垮落步距l[17]分别为
$$ \left\{ \begin{gathered} l = h\sqrt {{{2{R_{\rm{T}}}}}/{{{q_2}}}} \\ L = l\left( { {l}/{S} + \sqrt {{{{l^2}}}/{{{S^2}}} + {3}/{2}} } \right) \\ \end{gathered} \right. $$ (3) 式中：h为基本顶岩层厚度；RT为基本顶抗拉强度；q2为基本顶载荷；S为基本顶沿工作面悬露长度。
岩块C水平方向受力取0，可得
$$ {T_{\rm{B}}} = {T_{\rm{C}}} = \dfrac{{{q_2}{L^2}}}{{2(h - \Delta {S_{\rm{ B}}})}} $$ (4) 式中：TB为岩块B在$ {B}' $处所受水平推力；TC为岩块C在$ {C}' $处所受水平推力；$ \Delta {S}_{\rm{ B}} $为岩块B在$ {B}' $处的下沉量。
岩块C垂直方向受力取0，可得
$$ {N_{\rm{B}}} + {F_{\rm{G}}} = {N_{\rm{B}}} + {K_{\rm{G}}}L = {N_{\rm{C}}} + {q_2}L $$ (5) 式中：$ {N}_{\rm{B}} $，$ {N}_{\rm{C}} $分别为岩块B，C所受剪切力；FG为采空区矸石对基本顶的支撑力；$ {K}_{\rm{G}} $为采空区矸石支撑系数。
$ {B}' $点力矩取0，可得
$$ {M_{\rm{B}}} + {T_{\rm{C}}}(h - \Delta {{S}_{\rm{ C}}}) + {F_{\rm{G}}}L/2 - {N_{\rm{C}}}L - {q_2}{L^2}/2 = 0 $$ (6) $$ {N_{\rm{C}}} = \dfrac{{{M_{\rm{B}}} + {T_{\rm{B}}}(h - \Delta {{S}_{\rm{ C}}}) - {q_2}{L^2}/2}}{L} + {F_{\rm{G}}}/2 $$ (7) $$ {N_{\rm{B}}} = \dfrac{{{M_{\rm{B}}} + {T_{\rm{B}}}(h - \Delta {{S}_{\rm{ C}}}) + {q_2}{L^2}/2}}{L} - {F_{\rm{G}}}/2 $$ (8) 式中MB为岩块B在$ {B}' $处的残余弯矩。
对于岩块B，$ {A}' $点力矩取0，可得
$$ \begin{split} &{M_{\rm{A}}} + {M_0} + {f_{\rm{t}}}(a + b + {x_0}) + \int_0^{{x_0}} \sigma ({x_0} - x){\mathrm{d}}x +{T_{\rm{B}}}(h - \Delta {{S}_{\rm{ B}}}) - \\ &\qquad {M_{\rm{B}}} - {q_2}{l^2}/2 - {q_1}({x_0} + a + b)^2/2 -{N_{\rm{B}}}l = 0 \\[-5pt] \end{split} $$ (9) 式中：MA为岩块A在$ {A}' $处的残余弯矩；M0为直接顶对基本顶的抗弯弯矩；ft为巷旁支护阻力；a为巷旁支护体宽度；b为巷道宽度；x为模型中任一点的横坐标；q1为直接顶载荷。
联立式（3）、式（4）、式（8）、式（9）得
$$ \begin{split} {f_{\rm{t}}} =& \left\{ {} \right.2{M_{\rm{B}}} + {q_2}{L^2} + {q_1}{\left( {{x_0} + a + b} \right)^2}/2 + \\ & {q_2}{L^2}\left( {\Delta {{S}_{\rm{ B}}} - \Delta {{S}_{\rm{ C}}}} \right)/\left[ {2\left( {h - \Delta {{S}_{\rm{ B}}}} \right)} \right] - {F_{\rm{G}}}L/2 - {M_{\rm{A}}} - \\ & {M_0} - \int_0^{{x_0}} {\sigma \left( {{x_0} - x} \right){\rm{d}}x} \left. {} \right\}/ \left( {{x_0} + b + a/2} \right) \end{split} $$ (10) 式中$ \Delta {S}_{\rm{ C}} $为岩块C在$ {C}' $处的下沉量。
2.3 巷道底板受力分析
顶板垮落后，底板应力重新分布。为了简化计算，假设煤帮弹性区、塑性区的垂直应力呈线性分布，沿工作面走向方向取剖面，建立沿空留巷底板力学模型，如图4所示。fg为顶板破断岩块对底板的作用力；xm为顶板旋转下沉区宽度；fm为冒落矸石的重力；x1为弹性压缩区宽度；f0，f1分别为塑性区、弹性压缩区煤体对底板的作用力。
实体煤塑性区作用在底板的力为
$$ {f_0} = {{\left( {k - 1} \right)\gamma H}}\left( {{x_0} - x} \right)/{{{x_0}}} \quad - {x_0} \leqslant x \leqslant 0 $$ (11) 实体煤的弹性压缩区和塑性区共同承担了上覆岩层载荷和采动引起的应力重新分布，因此$ \left({x}_{1}+ {x}_{0}\right)\left(k+1\right)\gamma H/2=\gamma H{S}_{ 0}/2 $，S0为工作面长度，则
$$ {x_1} = {{{S_{ 0}}}}/{{(k + 1)}} - {x_0} $$ (12) 实体煤弹性压缩区作用在底板的力为
$$ {f_1} = {{\left( {k - 1} \right)\gamma H}}\left( {x - {x_1}} \right)/{{({x_1} - {x_0})}} \quad - {x_0} - {x_1} \leqslant x \leqslant - {x_0} $$ (13) 直接顶垮落矸石对底板的作用力为
$$ {f_{\text{m}}} = {h_1}{\gamma _1} $$ (14) 式中：h1为直接顶岩层厚度；γ1为直接顶岩层容重。
上方基本顶岩块C垮落后，沿倾向逐渐将矸石堆压实。顶板旋转下沉区宽度[18]为
$$ {x_{\rm{m}}} = h\cot\; \delta + L $$ (15) 式中δ为基本顶的破断角[19]。
$$ \delta=45^{\circ}-\dfrac{1}{2}\varphi_2+\dfrac{1}{2}\arctan l\sqrt{\dfrac{R_{\rm{T}}}{q_2}} $$ (16) 式中φ2为基本顶内摩擦角。
顶板破断下沉岩块对底板的作用力为
$$ {f_{\rm{g}}} = {{2{F_{\rm{G}}}}}\left[ {x - \left( {L - {x_0}} \right)} \right]/{{x_{\rm{m}}^2}} $$ (17) 2.4 巷道底板变形分析
引用等效载荷概念[20]，以消除原岩应力$\gamma H$对底板变形的影响。建立沿空留巷底板等效载荷分布力学模型，如图5所示。
![]()
图 5 沿空留巷底板等效载荷分布力学模型Figure 5. Mechanical model of equivalent load distribution on the floor of gob-side entry retaining在半无限平面边界上，点I所受应力为原岩应力，设垂直位移为0，将该点设为基点，则分布载荷q（x）在边界上点J（横坐标为xJ）处的垂直位移[21-22]为
$$ {\mathrm{d}}{U_{{J}}} = \dfrac{{2q\left( x \right)}}{{{\text{π}} {E_{\rm{d}}}}}\ln \dfrac{s}{\rho }{\mathrm{d}}\rho $$ (18) 式中：UJ为点J处的底鼓量；Ed为巷道底板岩层弹性模量；s为基点I到q（x）的距离；ρ为点J到q（x）的距离。
将不同分布载荷在J处引起的垂直位移相加，求得J处的底鼓量：
$$ \begin{split} {U_{{J}}} =& \dfrac{2}{{{\text{π}} {E_{\rm{d}}}}}\Bigg[\int_{ - \left( {{x_0} + {x_1}} \right)}^{ - {x_0}} {\left( {{f_1} - \gamma H} \right)\ln \dfrac{s}{\rho }{\rm{d}}x} + \int_{ - {x_0}}^0 {\left( {{f_0} - \gamma H} \right)} \\ &\ln \dfrac{s}{\rho }{\rm{d}}x +\int_b^{b + a} {\left( {{f_{\rm{t}}} - \gamma H} \right)\ln \dfrac{s}{\rho }{\rm{d}}x} + \int_{b + a}^{L - {x_0}} {({f_{\rm{m}}} - } \gamma H) \\ &\ln \dfrac{s}{\rho }{\rm{d}}x +\int_{L - {x_0}}^{L - {x_0} + {x_{\rm{m}}}} {\left( {{f_{\rm{m}}} + {f_{\rm{g}}} - \gamma H} \right)\ln \dfrac{s}{\rho }{\rm{d}}x} \Bigg]\\[-5pt] \end{split} $$ (19) 3. 切顶卸压对沿空留巷围岩结构影响
3.1 切顶卸压围岩结构力学分析
通过预爆破切顶，基本顶沿切顶缝断裂，侧向悬臂梁长度大幅减小，在巷道上方形成短臂梁结构[23]。由于直接顶厚度极薄，垮落后形成的矸石堆不足以充填整个采空区并支撑上覆岩层，所以采空区上方破断基本顶沿切缝大幅下沉并压实下方矸石堆。切顶后沿空留巷围岩结构如图6所示。
![]()
图 6 切顶后沿空留巷围岩结构Figure 6. Surrounding rock structure of gob-side entry retaining after roof cutting对切顶后沿空留巷顶板力学模型作如下假设：① 切顶角度为90°，简化力学模型。② 采空区侧基本顶沿切缝直接断裂垮落，岩块下沉时旋转角度为0。③ 垮落后的矸石堆积在采空区，对下沉基本顶起到支撑作用[24]。④ 忽略直接顶、基本顶及更上位岩层之间的剪切力。⑤ 基本顶上覆岩层载荷均匀施加在基本顶上。⑥ 巷内支护的变形忽略不计。⑦ 实体煤弹塑性区域及煤层底板所受应力在切顶前后的变化较覆岩整体的运移程度小，故忽略不计。
根据假设，构建切顶后沿空留巷力学模型，如图7所示。$ {T}_{\rm{B}}' $为岩块A在$ {B}' $处所受水平推力；$ {T}_{\rm{C}}' $为岩块B在$ {C}' $处所受水平推力；$ {N}_{\rm{B}}' $，$ {N}_{\rm{C}}' $分别为岩块A，B受到的剪切力；$ {M}_{\rm{A}}' $为岩块A在$ {A}' $处产生的弯矩；$ {M}_{\rm{B}}' $为岩块B在$ {B}' $处的残余弯矩；$ {f}_{\rm{t}}' $为切顶后巷旁支护阻力；$ {F}_{\rm{G}}' $为切顶后采空区矸石对基本顶支撑力。
![]()
图 7 切顶后沿空留巷力学模型Figure 7. Mechanical modeling of gob-side entry retaining after roof cutting根据静力平衡可得
$$ {N'_{\rm{C}}} = \dfrac{{{M'_{\rm{B}}} + {{T}'_{\rm{C}}}(h - \Delta {{S}'_{\rm{C}}}) - q{L^2}/2}}{L} + {F'_{\rm{G}}}/2 $$ (20) $$ {N'_{\rm{B}}} = \dfrac{{{M'_{\rm{B}}} + {{T}'_{\rm{C}}}(h - \Delta {{S}'_{\rm{C}}}) + q{L^2}/2}}{L} - {F'_{\rm{G}}}/2 $$ (21) $$ {T'_{\rm{C}}} = \dfrac{{{q_2}{L^2}}}{{2(h - \Delta {S'_{\rm{C}}})}} $$ (22) 式中$ \Delta {S}_{\rm{ C}}' $为岩块B在$ {C}' $处的下沉量。
$$ {\begin{split} {f'_{\rm{t}}} =& [{M'_{\rm{B}}}(L + {x_0} + a + b)/L + {q_2}{({x_0} + a + b)^2}/2 + {q_2}L({x_0} +\\ & a + b)/2 + {q_1}{({x_0} + a + b)^2}/2 - {{F}'_{\rm{G}}}({x_0} + a + b)/2 -\\ & {M'_{\rm{A}}} - {M_0} - \int_0^{{x_0}} \sigma ({x_0} - x){\rm{d}}x]/({x_0} + b + a/2) \end{split}} $$ (23) 3.2 切顶卸压巷道底板变形分析
切顶后沿空留巷底板等效载荷分布力学模型如图8所示。
![]()
图 8 切顶后沿空留巷底板等效载荷分布力学模型Figure 8. Mechanical model of equivalent load distribution on the floor of gob-side entry retaining after roof cutting切顶后顶板破断岩块对底板作用力为
$$ {f'_{\rm{g}}} = {F'_{\rm{G}}} = {K'_{\rm{G}}}L $$ (24) 式中$ {K}_{\rm{G}}' $为切顶后采空区矸石支撑系数。
底板上M点的底鼓量为
$${\begin{split} {U'_{\rm{M}}} =& \dfrac{2}{{{\text{π}} {E_{\rm{d}}}}}\Bigg[\int_{ - \left( {{x_0} + {x_1}} \right)}^{ - {x_0}} {\left( {{f_1} - \gamma H} \right)\ln \dfrac{s}{\rho }{\rm{d}}x} + \int_{ - {x_0}}^0 {\left( {{f_0} - \gamma H} \right)} \ln \dfrac{s}{\rho }{\rm{d}}x + \\ & \int_0^{b + a} {\left( {{f'_{\rm{t}}} - \gamma H} \right)\ln \dfrac{s}{\rho }{\rm{d}}x} + \int_{b + a}^{b + a + {x_{\rm{m}}}} {\left( {{f_{\rm{m}}} + {f'_{\rm{g}}} - \gamma H} \right)\ln \dfrac{s}{\rho }{\rm{d}}x} \Bigg] \\ \end{split}} $$ (25) 4. 切顶卸压底鼓数值模拟研究
4.1 模型的建立
采用Mohr-Coulomb本构模型，从现场获取巷道围岩岩体试样，按照相关要求制备成相应的标准试件。各岩层物理力学参数见表1。
表 1 各岩层物理力学参数Table 1. Physical and mechanical parameters of each rock formation岩性 弹性模
量/GPa抗拉强
度/MPa内摩擦
角/（°）泊松比 容重/
（kN·m−3）黏聚
力/MPa细砂岩 42 6.50 25 0.16 27.0 5.4 粉细砂岩 18 2.35 31 0.21 26.0 3.8 中砂岩 12 2.11 28 0.22 26.2 3.3 泥岩 6 1.47 24 0.21 22.5 2.4 1煤 0.8 1.20 21 0.35 13.8 1.8 砂质泥岩 9 2.91 24 0.23 25.2 2.8 根据地质资料建立立体模型，模拟在上一工作面回采完毕后，接替回采1615A工作面时，切顶卸压对巷道底板变形的控制效果。
4.2 沿空留巷巷道演化特征
4.2.1 切顶卸压巷道围岩破坏分布特征及应力分析
沿走向取剖面，切顶卸压前后工作面处破坏分布特征如图9所示。可看出工作面回采时采空区上方形成弹塑性破坏区域，且随工作面的推进逐渐向高位岩层发育，由破坏形态可知采空区上覆中位岩层逐渐趋于稳定。巷道实体煤侧及顶部围岩在切顶卸压后，破坏区域显著减小。随着工作面的推进，采用切顶卸压技术可有效缩小巷道实体煤侧面及顶部的破坏区域，维持巷道围岩结构稳定。
![]()
图 9 切顶卸压前后工作面破坏分布特征对比Figure 9. Comparison of damage distribution characteristics of the working face before and after roof cutting and pressure relief沿走向取剖面，切顶卸压前后工作面处垂直应力如图10所示。可看出：切顶卸压前，巷道实体煤侧出现应力集中现象，应力峰值随着工作面推进由19.22 MPa增加至23.13 MPa，巷旁支护阻力峰值为5.75 MPa，巷道底板中部应力峰值为9.77 MPa，对巷道围岩稳定造成严重影响；切顶卸压后，应力集中向巷道顶板上方及深部转移，远离巷道围岩，应力峰值由19.92 MPa增加至28.20 MPa，巷旁支护阻力峰值减少至2.58 MPa，巷道底板中部应力峰值为7.25 MPa，巷道顶板区域所受应力显著减小。采用切顶卸压技术可有效优化巷道围岩应力结构，巷旁支护阻力降幅为56.14%，底板最大应力平均降幅为25.78%。
![]()
图 10 切顶卸压前后工作面垂直应力对比Figure 10. Comparison of vertical stress on the working face before and after roof cutting and pressure relief4.2.2 切顶卸压巷道底鼓分析
对整条巷道底板变形量进行监测，结果如图11所示。可知切顶前，工作面超前处巷道底板变形量在503～917 mm波动，均值为664 mm；切顶后，工作面超前处巷道底板变形量在204～409 mm波动，均值为305 mm，较切顶前下降54.07%。沿空留巷切顶卸压有效缓解了沿空留巷底鼓。
![]()
图 11 工作面推进切顶卸压前后巷道底鼓对比Figure 11. Comparison of floor heave before and after roof cutting and pressure relief5. 工程应用
预裂切缝钻孔深12 m，切缝角度为15°。采用双向聚能爆破预裂技术，切缝距煤帮100 mm，切缝孔间距为500 mm。特质聚能管外径为42 mm，内径为36.50 mm，管长1 500 mm。炮空底部装药量为3～5卷，炮孔处为1～2卷，炸药规格为ϕ35×330 mm/卷。现场炮孔布置如图12所示。
结合现场数据对巷道底鼓解析解进行验证。基本参数：hc=6.5 m，h=13.6 m，px=0.1 MPa，φ=24°，H=600 m，γ=25 kN/m3，c=2.4 MPa，k=2.6，S0=200 m，Ed=9.0 GPa，η=0.9，KP0=1.10，a=1 m，b=5 m。
其他参数设置：直接顶及基本顶载荷根据q=γh确定；垮落长度L=20 m；岩梁断裂处残余弯矩为0；$ \Delta {S}_{\rm{ B}}=\Delta {S}_{\rm{ C}} $；实体煤塑性区宽度x0=10.3 m；切顶前后采空区矸石对基本顶支撑力FG=5.237 MN，$ {F}_{\rm{G}}' $=10.532 MN；切顶前后巷旁支护阻力ft=5.564 5 MN，$ {f}_{\rm{t}}' $=2.194 2 MN。
将上述参数代入式（19）、式（25），可得切顶前后巷道底鼓量$ {U}_{\rm{M}}=-709.345\;1\;\mathrm{m}\mathrm{m} $，$ {U}_{\rm{M}}'=320.965\;8\;\mathrm{m}\mathrm{m} $。
切顶前后沿空留巷底鼓情况如图13所示。可看出沿空留巷切顶后，巷道底鼓量减小，切顶卸压有效改善巷道底板破坏情况。
![]()
图 13 切顶前后沿空留巷底鼓情况Figure 13. Condition of the floor heave in the gob-side entry retaining before and after roof cutting6. 结论
1） 针对工作面现场情况，运用给定变形理论，构建了沿空留巷顶板岩层力学模型，推导出切顶卸压前后巷旁支护阻力的计算方法。
2） 运用弹性力学知识，结合底板各区域所受载荷，推导出切顶卸压前后巷道底鼓计算公式。分析得出巷旁煤帮弹塑性区、巷道支护体及顶板下沉区底板所受载荷共同影响巷道底鼓量大小。
3） 切顶卸压后，应力集中现象向巷道顶板上方及深部转移，巷道底板最大应力平均降幅为25.78%，巷旁支护阻力平均降幅为56.14%；厚硬顶板沿空留巷底鼓量由709.345 1 mm降至320.965 8 mm。切顶卸压技术可以优化巷道围岩应力结构，抑制巷道底鼓，有效改善底板破坏情况。
-
![]()
图 5 L=0时所有模型的5种截面位置所对应的风流场云图
Figure 5. Cloud plot of wind fields corresponding to the five cross-sectional positions of all models at L =0 m
![]()
图 6 L=0.5 m时所有模型的5种截面位置所对应的风流场云图
Figure 6. Cloud plot of wind fields corresponding to the five cross-sectional positions of all models at L =0.5 m
![]()
图 7 L=1 m时所有模型的5种截面位置所对应的风流场云图
Figure 7. Cloud plot of wind fields corresponding to the five cross-sectional positions of all models at L=1 m
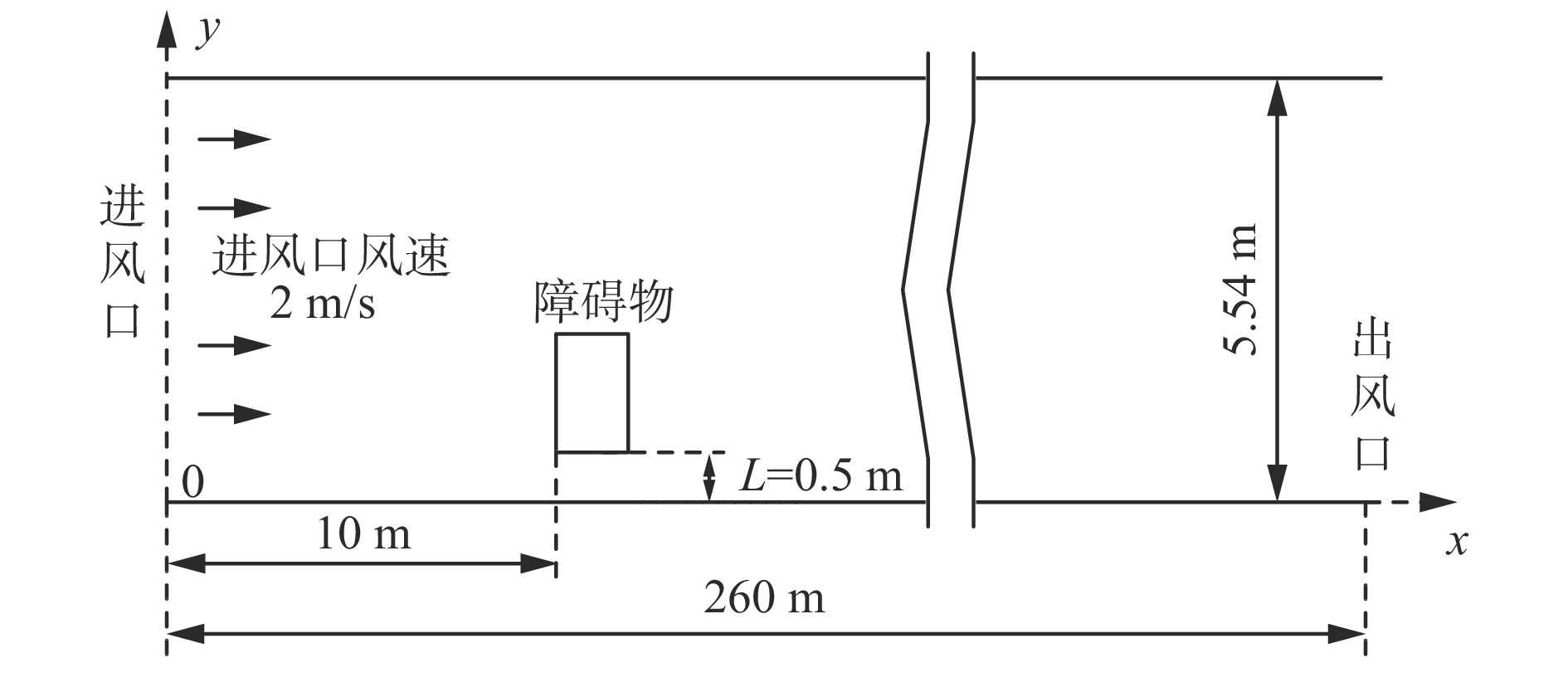
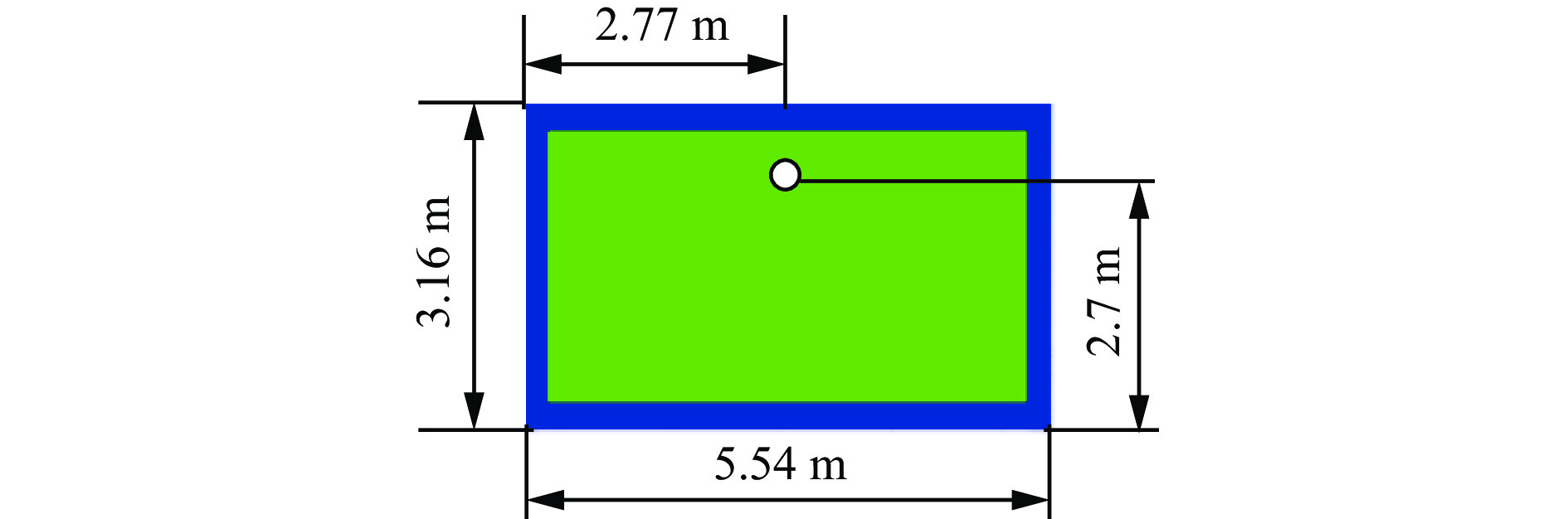
表 1 11218回风巷截面参数信息
Table 1 11218 return air roadway parameter information
高度/m 宽度/m 周长/m 断面积/m2 进风口风速/（m·s−1） 3.16 5.54 17.4 17.51 2 
下载: 导出CSV
表 2 障碍物信息
Table 2 Obstacle information
l/m b/m h/m 障碍物1 1 1 1 障碍物2 2 1 1 障碍物3 1 1 0.5 障碍物4 2 1 0.5 障碍物5 1 0.5 1 障碍物6 2 0.5 1
下载: 导出CSV
-
[1] 袁亮. 煤及共伴生资源精准开采科学问题与对策[J]. 煤炭学报,2019,44(1):1-9. YUAN Liang. Scientific problem and countermeasure for precision mining of coal and associated resources[J]. Journal of China Coal Society,2019,44(1):1-9.
[2] 刘剑,李雪冰,宋莹,等. 无外部扰动的均直巷道风速和风压测不准机理实验研究[J]. 煤炭学报,2016,41(6):1447-1453. LIU Jian,LI Xuebing,SONG Ying,et al. Experimental study on uncertainty mechanism of mine airvelocity and pressure with non-external disturbance[J]. Journal of China Coal Society,2016,41(6):1447-1453.
[3] 李雪冰,刘剑,秦洪岩,等. 湍流脉动影响下巷道平均风速单点统计测量方法[J]. 华北科技学院学报,2018,15(2):1-9. LI Xuebing,LIU Jian,QIN Hongyan,et al. Method for air velocity measurement with single-point under the influence of turbulent fluctuation[J]. Journal of North China Institute of Science and Technology,2018,15(2):1-9.
[4] 丁翠,何学秋,聂百胜. 矿井通风巷道风流分布“关键环”数值与实验研究[J]. 辽宁工程技术大学学报(自然科学版),2015,34(10):1131-1136. DING Cui,HE Xueqiu,NIE Baisheng. Numerical and experimental research on "key ring" distribution of ventilation in mine tunnels[J]. Journal of Liaoning Technical University(Natural Science),2015,34(10):1131-1136.
[5] 杨宇,王毅. 煤矿井下拱形巷道低风速区风速分布的风洞模拟[J]. 煤炭技术,2017,36(6):42-45. YANG Yu,WANG Yi. Wind tunnel simulation on wind speed sistribution of low speed zone in coal mine arch roadway[J]. Coal Technology,2017,36(6):42-45.
[6] 潘竞涛. 基于最小二乘法的风速传感器测量值推导巷道平均风速[J]. 煤炭技术,2018,37(1):213-215. PAN Jingtao. Derivation of average wind speed of roadway from wind sensors measurements based on least square method[J]. Coal Technology,2018,37(1):213-215.
[7] 李亚俊,李印洪,吴洁葵,等. 巷道断面风流分布规律试验研究[J]. 有色金属(矿山部分),2019,71(5):102-104,110. LI Yajun,LI Yinhong,WU Jiekui,et al. Experimental study on air flow distribution law of the roadway[J]. Nonferrous Metals(Mining Section),2019,71(5):102-104,110.
[8] 宋莹,王东,郭欣,等. 突扩巷道流场风流分布特征的PIV实验研究[J]. 中国安全生产科学技术,2017,13(6):86-91. SONG Ying,WANG Dong,GUO Xin,et al. Experimental study on airflow distribution characteristics of flow field in sudden enlarged roadway based on PIV[J]. Journal of Safety Science and Technology,2017,13(6):86-91.
[9] 张浪. 巷道测风站风速传感器平均风速测定位置优化研究[J]. 煤炭科学技术,2018,46(3):96-102. ZHANG Lang. Optimized study on location to measure average air velocity with air velocity sensor in wind measuring station of underground mine[J]. Coal Science and Technology,2018,46(3):96-102.
[10] 张士岭. 煤矿通风巷道断面风速测定与变化规律研究[J]. 矿业安全与环保,2019,46(4):17-20. ZHANG Shiling. Study on measurement and change law of wind speed in cross section of coal mine ventilation roadway[J]. Mining Safety & Environmental Protection,2019,46(4):17-20.
[11] 鹿广利,武赞龙,赵剑锋. 不同拐弯角度下巷道内风流变化规律的数值模拟[J]. 矿业研究与开发,2019,39(12):116-121. LU Guangli,WU Zanlong,ZHAO Jianfeng. Numerical simulation on the change law of air flow in roadway with different turning nagles[J]. Mining Research and Development,2019,39(12):116-121.
[12] 张京兆,王艳,魏引尚,等. 入口形式对矩形巷道定点测风位置影响研究[J]. 矿业研究与开发,2021,41(6):154-157. ZHANG Jingzhao,WANG Yan,WEI Yinshang,et al. Study on the influence of inlet patterns on the fixed-point air velocity measurement location in a rectangular airway[J]. Mining Research and Development,2021,41(6):154-157.
[13] 李虎民,陈国庆,熊帅,等. 相对粗糙度对巷道定点测风位置影响的数值分析[J]. 矿业研究与开发,2021,41(9):113-117. LI Humin,CHEN Guoqing,XIONG Shuai,et al. Numerical analysis on the influence of relative roughness on the fixed-point air velocity measurement location in an roadway[J]. Mining Research and Development,2021,41(9):113-117.
[14] 盛典. 基于FLUENT的煤矿井下风门通风系统研究[J]. 煤炭技术,2022,41(5):149-151. SHENG Dian. Research on ventilation system of air door in coal mine based on FLUENT[J]. Coal Technology,2022,41(5):149-151.
[15] 葛启发,于润沧,翟建波,等. 基于FLUENT的进路式采场通风优化控制[J]. 矿业研究与开发,2017,37(12):111-116. GE Qifa,YU Runcang,ZHAI Jianbo,et al. Ventilation optimization and control of drift-type stope based on FLUENT[J]. Mining Research and Development,2017,37(12):111-116.
[16] 郭对明,李国清,侯杰,等. 基于FLUENT的深井掘进巷道局部通风参数优化[J]. 黄金科学技术,2022,30(5):753-763. GUO Duiming,LI Guoqing,HOU Jie,et al. Optimization of local ventilation parameters of deep mine excavation roadway based on FLUENT[J]. Gold Science and Technology,2022,30(5):753-763.
[17] 王春龙,程力. 基于FLUENT的三山岛金矿深部通风降温系统数值模拟研究[J]. 矿业研究与开发,2022,42(2):129-134. WANG Chunlong,CHENG Li. Numerical simulation of deep ventilation and cooling system in Sanshandao Gold Mine based on FLUENT[J]. Mining Research and Development,2022,42(2):129-134.
[18] 王波,宋玉彬,王鑫. 基于ANSYS Workbench的隔爆壳体目标驱动优化设计[J]. 工矿自动化,2015,41(12):70-72. WANG Bo,SONG Yubin,WANG Xin. Goal driven optimization design of flameproof shell based on ANSYS Workbench[J]. Industry and Mine Automation,2015,41(12):70-72.
[19] 时国庆,王德明,奚志林,等. 基于FLUENT对采空区氧气浓度场的数值模拟[J]. 煤炭科学技术,2009,37(6):76-79. DOI: 10.13199/j.cst.2009.06.81.shigq.028 SHI Guoqing,WANG Deming,XI Zhilin,et al. Numerical simulation of oxygen concentration distribution in gob areas based on FLUENT[J]. Coal Science and Technology,2009,37(6):76-79. DOI: 10.13199/j.cst.2009.06.81.shigq.028
[20] 王翰锋. 基于Fluent巷道断面平均风速点定位监测模拟研究[J]. 煤炭科学技术,2015,43(8):92-96. DOI: 10.13199/j.cnki.cst.2015.08.018 WANG Hanfeng. Simulation study on monitoring and measuring location of average air velocity in section of mine roadway based on Fluent[J]. Coal Science and Technology,2015,43(8):92-96. DOI: 10.13199/j.cnki.cst.2015.08.018
[21] 刘剑,李雪冰,陈廷凯,等. 矿井定常湍流脉动对通风阻力测试影响的理论分析[J]. 中国安全生产科学技术,2016,12(5):22-25. LIU Jian,LI Xuebing,CHEN Tingkai,et al. Theoretical analysis on influence of steady turbulence fluctuation on ventilation resistance measurement in mine[J]. Journal of Safety Science and Technology,2016,12(5):22-25.
-
期刊类型引用(3)
1. 李刚，刘航，迟国铭，石占山，范永君. 大柳塔煤矿沿空留巷柔模砼墙支护阻力核定及失稳判据研究. 工矿自动化. 2025(01): 145-155 . 
本站查看
2. 吕彦国. 深孔爆破切顶卸压沿空留巷支护技术研究. 建井技术. 2024(06): 1-7 . 百度学术
3. 孟国龙. 某深部煤层综采工作面切顶卸压留巷技术研究. 现代矿业. 2024(12): 48-51+59 . 百度学术
其他类型引用(0)
计量
- 文章访问数: 1060
- HTML全文浏览量: 53
- PDF下载量: 34
- 被引次数: 3
